Hauser's Algebraic Surface Gallery

by silviana amethyst

Reproductions in 3D of Herwig Hauser's fine raytraced surfaces, using real numerical cellular decomposition implemented in Bertini_real

Regarding how I printed these models, I have some advice here.
Click on the pictures to find out more about the surfaces.
Non-clickable pictures still need me to write a description for them. These are indicated with a ° next to the name of the surface.

Herwig Hauser gallery medley of 3d printed algebraic surfaces Tobel, Croissant, Kreuz, Stern

14 of the 72 remain to be printed as of July 2018. Many have been printed but not yet photographed or documented. This turned out to be a long project! I started it (informally) as I started implementing the surface decomposition routine of Bertini_real in Fall 2013. Each surface takes 15-30 minutes to writeup and post, and there's also the computation time, post-processing to make it printable, and print time. This project is truly a labor of love. I thank those who have helped me with it over the years.

Calyx
\(x^2+y^2 z^3 = z^4\)

Calypso
\(x^2+y^2 z = z^2\)

Columpius
\(x^3y + xz^3 +y^3z + z\)
\( + 7z^2 + 5z\)

Cube
\(x^6+y^6+z^6 = 1\)

Dattel °
\(3x^2+3y^2+z^2=1\)

Daisy °
\((x^2-y^3)^2 = (z^2-y^2)^3\)

Dingdong
\(x^2+y^2+z^3=z^2\)

Distel
\(x^2+y^2+z^2 + 1000(x^2+y^2) \)
\((x^2+z^2)(y^2+z^2) = 1\)

Durchblick
\( x^3 y+ xz^3 +y^3z+z^3+5z \)

Eistute °
\( (x^2+y^2)^3 = 4x^2y^2(z^2+1) \)

Eve
\( \frac{1}{2}x^2+2xz^2+5y^6+15y^4+\frac{1}{2}z^2 = 15y^5 +5y^3 \)

Flirt °
\( x^2-x^3+y^2+y^4+z^3-10z^ 4 \)

Geisha
\( x^2yz+x^2z^2 = y^3z+y^3 \)

Harlekin °
\(x^3z+10x^2y+xy^2+yz^2 = z^3 \)

Helix
\( 6x^2-2x^4 = y^2z^2 \)

Herz °
\( y^2+z^3-z^4-x^2z^2 \)

Himmel und Holle
\( x^2-y^2z^2 \)

Kolibri
\( x^3+x^2z^2 - y^2 \)

Leopold
\( 1000 x^2y^2z^2 + 3x^2+3y^2+z^2=1 \)

Octdong °
\( x^2+y^2+z^4 = z^2 \)

Plop
\( x^2 + (z+y^2)^3 \)

Seepferdchen
\( x^4-2.5x^2y^3 -xz^3 +y^6 -y^2z^3 \)

Sofa °
\( x^2+y^3+z^5 \)

Solitude
\( x^2yz+xy^2+y^3+y^3z = x^2z^2 \)

Suss
\( (x^2+\frac{9}{4}y^2 + z^2-1)^3 - x^2z^3 - \)
\( \frac{9}{80}y^2z^3 \)

Tanz °
\( x^4-x^2-y^2z^2 \)

Taube °
\( 256z^3-128x^2z^2+16x^4z \)
\( +144 xy^2z-4x^3y^2-27y^4 \)

Quaste °
equation not given

Spitz °
\( (y^3-x^2-z^2)^3 = 27x^2y^3z^2 \)

Tobel
\( x^3z+x^2+yz^3+z^4 = 3xyz \)

Vis a vis °
\( x^2-x^3+y^2+y^4+z^3-z^4 \)

Wedeln °
\( x^3=y(1-z^2)^2 \)

Windkanal °
\( -x^2+y^4+z^4-xyz = 100 \)

Xano °
\( x^3+z^3=yz^2 \)

Zitrus °
\( x^2+z^2+y^3(y-1)^3 \)

Croissant °
equation not given

Dromedar °
\( x^4-3x^2+y^2+z^3 \)

Zeppelin °
\( xyz+yz+2z^5 \)

Zweiloch °
\( x^3y+xz^3+y^3z + z^3+7z^2+5z \)

Michelangelo °
\( x^2+y^4+y^3z^2 \)

Stern °
\( 400(x^2y^2+y^2z^2 +x^2z^2) + (x^2+y^2+z^2 -1)^3 \)

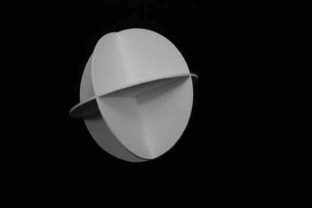
Mobius °
equation not given

Sphare °
\( x^2+y^2+z^2=1 \)

Limao °
\( x^2-y^3z^3 \)

Torus °
\( (x^2+y^2\)
\( +z^2+R^2-r^2)^2 \)
\( = R^2(x^2+y^2) \)

Whitney °
\( x^2-y^2z \)

Buggle °
\( x^4y^2+y^4x^2-x^2y^2 + z^6 \)

Zylinder °
\( y^2+z^2=1 \)

Diabolo °
\( x^2=(y^2+z^2)^2 \)

Dullo °
\( (x^2+y^2+z^2)^2\)
\( -(x^2+y^2) \)

Miau °
\( x^2yz+x^2z^2\)
\( +2y^3z+3y^3 \)

Trichter °
\( x^2+z^3 \)
\( = y^2z^2 \)

Nepali °
\( (xy-z^3-1)^2 + (x^2+y^2-1)^3 \)

Pilzchen °
\( (z^3-1)^2+(x^2+y^2-1)^3 \)

Subway °
\( x^2y^2+(z^2-1)^3 \)

Polsterzipf
\( (x^3-1)^2+(y^3-1)^2+(z^2-1)^3 \)

Crixxi °
\( (y^2+z^2-1)^2 \)
\(+ (x^2+y^2-1)^3 \)

Berg °
\( x^2+y^2z^2 + z^3 \)

Gupf °
\( x^2+y^2+z \)

Kegel °
\( x^2+y^2-z^2 \)

Wigwam
\( x^2+y^2z^3 \)

Tuelle
\( yz(x^2+y-z) \)

Pipe °
\( x^2-z \)

Fanfare °
\( -x^3+z^2+y^2 \)

Kreuz
\( xyz \)

Spindel
\( x^2+y^2-z^2=1 \)

Twilight
\( (z^3-2)^2+\)
\((x^2+y^2-3)^3 \)

Ufo °
\( z^2-x^2-y^2=1 \)

Wendel °
equation not given

Zeck
\( x^2+y^2-z^3(1-z)\)

Sattel
\( x^2+y^2z + z^3\)

Schneeflocke °
\( x^3+y^2z^3+yz^4\)

I currently do the photography using a nylon photo booth, some spotlights, and a Canon Rebel xsi camera. If you identify ways for me to improve my photography, please email me and share! I want to take the best pictures I can possibly produce, and welcome the advice of others!

I have posted * low-res thumbnails on the main page, and medium quality copies without watermark on the focused pages, as a service to the mathematical and broader community. I retain all originals -- if you would like to use an original for a publication, please email me.